Multiplizieren von Summen und Differenzen: Beide Faktoren sind zweigliedrige Ausdrücke
Beispiel:
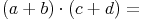
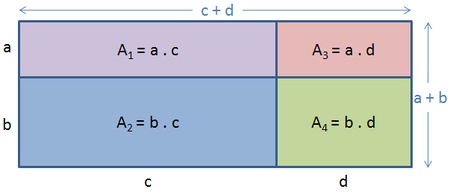
Wir berechnen den Flächeninhalt dieser Figur auf 2 verschiedenen Arten:
Möglichkeit 1:Wir berechnen den Flächeninhalt der gesamten Figur auf einmal:
A = Länge mal Breite

Wir berechnen die Flächeninhalte der vier unterteilten Flächen und addieren diese:
A = Länge mal Breite
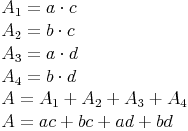
Beide Berechnungen führen zum selben Ergebnis, deshalb kann man die beiden Ergebnisse gleichsetzen:
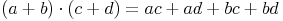
Multiplikation von Summen, wenn beide Faktoren zweigliedrige Ausdrücke sind:
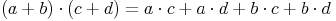
Beispiel:

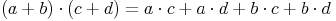
Beispiel:

Multiplikation von Summen und Differenzen, wenn beide Faktoren zweigliedrige Ausdrücke sind:
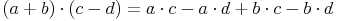
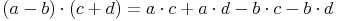

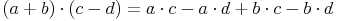
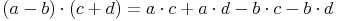

- Dieser Artikel hat mir geholfen. das half mir
- ... leider nicht ... leider nicht
- Kommentar Kommentar